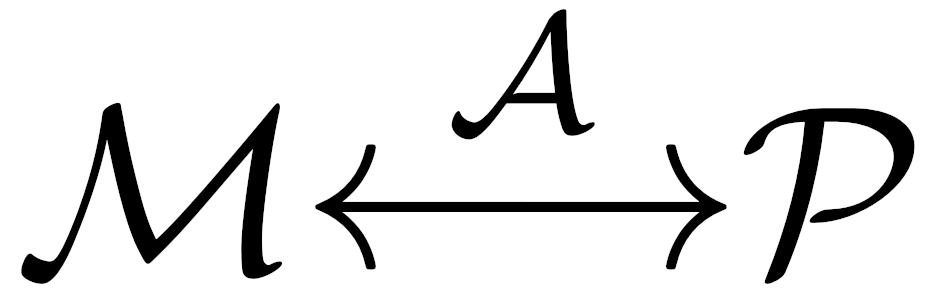Meeting Notes 2025-04-08

This Week’s Finds

No finds this week.

 

Focus Session

Speaker: Joshua
Title: Representations & Associated Vector Bundles: Fundamental & Adjoint

 

1. Overview

• This talk examines how the fundamental and adjoint representations of a Lie group \(G\) are used to build associated vector bundles in physics.
• Goals:
  1. Define the fundamental representation \(\rho_f\) and the adjoint representation \(\rho_{\rm adj}\) of \(G\).
  2. Construct associated bundles \(E = P \times_\rho V\) and describe how matter and gauge fields transform.

 

2. Main Content

1. Fundamental Representation
   • Definition: \(\rho_f: G \to GL(V)\) on a vector space \(V\) (e.g.\ \(\mathbb{C}^n\) for \(SU(n)\)).
   • Associated bundle: \(E_f = P \times_{\rho_f} V\), where \(P\) is a principal \(G\)-bundle.
   • Matter fields are sections \(\phi: M \to E_f\) and transform as \(\phi(x)\mapsto \rho_f\bigl(g(x)\bigr)\,\phi(x)\) under gauge transformation \(g:M\to G\).
2. Adjoint Representation
   • Definition: \(\rho_{\rm adj}: G \to GL\bigl(\mathfrak{g}\bigr)\) given by \(\rho_{\rm adj}(g)(X)=gXg^{-1}\) or \(\mathrm{Ad}_g(X)\).
   • Adjoint bundle: \(\mathrm{ad}(P) = P \times_{\mathrm{Ad}} \mathfrak{g}\) with fiber the Lie algebra \(\mathfrak{g}\).
   • Gauge fields (connections) are 1-forms \(A\in \Omega^1\bigl(M,\mathrm{ad}(P)\bigr)\) transforming as
     \(A \mapsto g\,A\,g^{-1} + g\,\mathrm{d}g^{-1}.\)
3. Fields & Transformations
   • Matter fields in \(E_f\) carry charge according to \(\rho_f\) (e.g.\ quark fields in the fundamental of \(SU(3)\)).
   • Gauge bosons correspond to sections of \(\mathrm{ad}(P)\) and transform in the adjoint rep.
   • Coupling term example: \(\bar\psi\,\gamma^\mu\,A_\mu\,\psi\) respects both representations.

 

3. Discussion Points

• How do different choices of the representation \(\rho_f\) affect matter field quantum numbers?
• Interpretation of the adjoint bundle curvature \(F \in \Omega^2(M,\mathrm{ad}(P))\) in terms of field strength.
• Extension to higher-dimensional or spinor bundles via spin representations.

 

Up Next

Next Talk:

• Alice on Characteristic Classes & Chern–Weil Theory

 

References

1. Kobayashi & Nomizu, Foundations of Differential Geometry, Vol. I, Ch. II.5
2. Nakahara, Geometry, Topology and Physics.
3. Representation Theory (Wikipedia)
4. Associated Bundle (Wikipedia)